- This page is about the ELL that solves edges preserving corners, for the method that does not, see LL edges first
Edges of Last Layer, or normally ELL, is a method that solves the last layer edges of the 3x3x3 in one algorithm as the last step in the solution, normally after performing CLL to solve the last layer corners. There are 29 cases in ELL, of these are three pure flips, four are EPLL and six cases are 3-cycles (besides the two in EPLL), all useful also for other methods, like BLD.
The chance of getting an ELL skip is after doing CLL is 1/96. The chance of getting an EPLL is 1/8. The probability of all edges being permuted is 1/12.
ELL is also used for a method of solving the last layer edges on larger cubes. Since there are 8 of each type of wing, though, ELL for larger cubes requires more than one look due to the large number of cases, see KBCM for a complete 3-look ELL for the 4x4x4 cube.
All ELL that are pure 3-cycles are used in the blindfold method TuRBo.
See also
External links
Intuitive
If you like to find algs for ELL in the MU-group, then knowing these short "proto algs" will help a lot:
- O = M' U M ... Orient
- O' = M' U' M ... Orient inverse
- P = M' U2 M ... Permute
If you combine these and also insert U-turns you can find optimal or near optimal algs for most ELLs
Example, 4-flip: P U2 O U2 P U' ... P U2 sets up for O that flips four edges (O' works the same in this case, choose the fastets), U2 P is restoration of the setup and last U is AUF.
2-look ELL
Use any ELL that orients what you currently got (3 cases) from the listings below and finnish it using EPLL (4 cases). Most OLLs that preserves corner orientation will probably also do for the first look but don't count on it, some may change corner permutation while orienting the edges.
Another way to solve ELL in 2 looks is to use L5E, but that is desigend for solving one more edge than this is so there will be nearly the same number of algs as full ELL (5+16).
ELL Algorithms
|
Note that all of these algorithms are written in the Western notation, where a lowercase letter means a double-layer turn and rotations are denoted by x, y, and z. (how to add algorithms)
Click on an algorithm (not the camera icon) to watch an animation of it.
|
The naming convention here is; wich edges are fliped if they where permuted and which EPLL would it be if all was correctly oriented.
Pure flips
2-flip (Adjacent)
|
2-flip (Opposite)
|
4-flip
|
|
EPLLs
Please do not add algs to the EPLL section, the ones here are just examples, all EPLLs are at the PLL-page, put your contributions there too, else we will end up having two separate lists and we don't want that.
U-PLL a
|
U-PLL b
|
Z-PLL
|
H-PLL
|
B edge solved (3-cycles)
The cases in this group, including U-PLL a and b, are used in the edge blindfold method TuRBo.
LF-flip U-PLL b
|

|
Name: Pure 3-cycle
Used in: ELL, BLD
Optimal moves: 9 HTM, 7 STM
|
|
RF-flip U-PLL a
|

|
Name: Pure 3-cycle
Used in: ELL, BLD
Optimal moves: 9 HTM, 7 STM
|
|
|
The following four cases are mirror + inverses of the first so you only need '1 alg' for all.
|
Mirror to the side and inverse in diagonal.
|
RL-flip U-PLL a
|

|
Name: Pure 3-cycle
Used in: ELL, BLD
Optimal moves: 10 HTM, 8 STM
|
|
RL-flip U-PLL b
|

|
Name: Pure 3-cycle
Used in: ELL, BLD
Optimal moves: 10 HTM, 8 STM
|
|
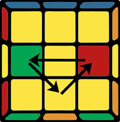
LF-flip U-PLL a
|
|
Name: Pure 3-cycle
Used in: ELL, BLD
Optimal moves: 10 HTM, 8 STM
|
|
RF-flip U-PLL b
|

|
Name: Pure 3-cycle
Used in: ELL, BLD
Optimal moves: 10 HTM, 8 STM
|
|
B edge in position but flipped
The first six cases in this group are the worst of ELL, and that counts for both recognition and solving :-/
4-flip U-PLL a
|

|
Name: 3-cycle 1-flip
Used in: ELL
Optimal moves: 13 HTM, 12 STM
|
|
4-flip U-PLL b
|

|
Name: 3-cycle 1-flip
Used in: ELL
Optimal moves: 13 HTM, 12 STM
|
|
|
The following four cases are mirror + inverses of the first so you only need '1 alg' for all.
|
Mirror to the side and inverse in diagonal.
|
BF-flip U-PLL a
|

|
Name: 3-cycle 1-flip
Used in: ELL
Optimal moves: 13 HTM, 10 STM
|
|
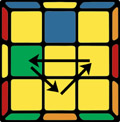
BF-flip U-PLL b
|
|
Name: 3-cycle 1-flip
Used in: ELL
Optimal moves: 13 HTM, 10 STM
|
|
BL-flip U-PLL a
|

|
Name: 3-cycle 1-flip
Used in: ELL
Optimal moves: 13 HTM, 11 STM
|
|
BR-flip U-PLL b
|

|
Name: 3-cycle 1-flip
Used in: ELL
Optimal moves: 13 HTM, 11 STM
|
|
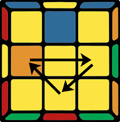
BR-flip U-PLL a
|
|
Name: 3-cycle 1-flip
Used in: ELL
Optimal moves: 13 HTM, 11 STM
|
|
BL-flip U-PLL b
|

|
Name: 3-cycle 1-flip
Used in: ELL
Optimal moves: 13 HTM, 11 STM
|
|
No edges in position
LF-flip Z-PLL
|
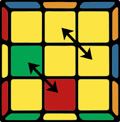
|
Name: Adjacent flip Z-PLL a
Used in: ELL
Optimal moves: 11 HTM, 11 STM
|
|
LB-flip Z-PLL
|

|
Name: Adjacent flip Z-PLL b
Used in: ELL
Optimal moves: 13 HTM, 13 STM
|
|
FB-flip Z-PLL
|

|
Name: Opposite flip Z-PLL a
Used in: ELL
Optimal moves: 14 HTM, ? STM
|
|
LR-flip Z-PLL
|

|
Name: Opposite flip Z-PLL b
Used in: ELL
Optimal moves: 14 HTM, ? STM
|
|
4-flip Z-PLL
|

|
Name: 4-flip Z-PLL
Used in: ELL
Optimal moves: 13 HTM, 11 STM
|
|
4-flip H-PLL
|

|
Name: 4-flip H-PLL
Used in: ELL
Optimal moves: 12 HTM, 12 STM
|
|
FR-flip H-PLL
|

|
Name: Adjacent flip H-PLL
Used in: ELL
Optimal moves: 10 HTM, 10 STM
|
|
FB-flip H-PLL
|

|
Name: Opposite flip H-PLL
Used in: ELL
Optimal moves: 15 HTM, 11 STM
|
|